Who needs maths? You.
I was just reading this article on BBC News about the problem we have in the UK of not enough people studying maths, and the general cultural issue of maths being seen as difficult and uncool to be good at.
I always thought it was weird that people have this attitude towards maths, at my house when I was little maths was always talked about as a fun interesting thing, and possibly as a result of that or possibly because of some mental aptitude I always found it really easy. I did Maths, Further Maths and Physics A-Levels because the amount of duplication on the courses meant it was much less work than three completely different A-Levels. And you got no essays to write.
The thing that really surprised me about that article is that you have proper adults, presumably out working in the real world, saying they don't use maths in the real world. Really? What jobs are they doing? Even if you aren't doing a mathematical job there are tons of areas where you use maths every day.
eg. in light of current housing market problems I have been making a few calculations around mortgages. A simple calculation might be if house prices drop by x% how much would I have to overpay my mortgage by to make sure I have paid off enough of the loan to be able to get a remortgage deal this time next year? (too much)
People must be working stuff like this out all the time. What difference will a fuel price rise of 5p make to my monthly fuel costs? How many people spend any time at all having to work with spreadsheets, performing computations on rows and getting complex reports out? Or have to create or read any kind of graph? That's all maths. Builders need to work out stuff like "how much cement will I need?", or "given that VAT is 17.5%, how much should I knock off for cash if I'm not going to declare this to the taxman?", or "how much of this weeks salary can I send back to Poland?". What kind of surcharge will I have to pay for my flight at the check-in desk if I weigh 220lbs, my bag is 33lbs and it's a 5$ surcharge per kilo for every kilo, or part thereof over 100 kilos.
Perhaps people don't realise how much maths they are using when doing stuff like that.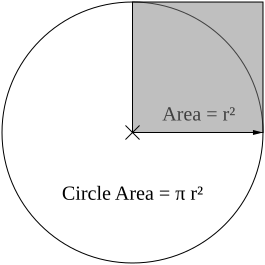
The other day I had to work out the difference in area between taking a circle for a "within x miles" calculation and just using a square.* I ended up having a discussion with a drunk friend of my brothers that evening. He actually said "When does anyone use something like geometry in the real world?".
Sometimes knowing a bit of maths and being able to work something out makes life easier, sometimes it's quite fun to be able to work something out. It can also save you money and money = work therefore maths = good.
*Answer is that the square is 4/π times larger, or the area of the circle is 79% of the area of the square.



10 comments:
Spotted on the BBC site:
If a=1 and b=1 then:
a^2 -b^2 =0, and
a^2 - ab =0, therefore:
a^2 - ab =a^2 -b^2, factorising:
a(a-b)=(a+b)(a-b), dividing by (a-b):
a=a+b
1=2
That's clever but wrong because they defined a = b. a^2 - ab = 0 defines that a = b
So a - b = 0
The line a(a-b)=(a+b)(a-b) therefore means
a*0 = a+b *0 which is always true for any value of a and b.
Once they factorise it they are dividing by 0, which you can't do.
I know. I thought you'd like it though.
Or it might make your head explode.
I'm not that hungover thankfully.
"The other day I had to work out the difference in area between taking a circle for a 'within x miles' calculation and just using a square."
WTF? Seriously? Such excitement, such excitement.
Checked you from Apolyton (OT).
I can hardly breach the subject without discussing everything, which is a good deal mare than we can read.
Math is useless, except when it's useful. Those exceptions are rare.
You blog regards applied math, which is fine, dandy, and "true enough". But we need pure mathematicians to create the new applied maths. The usefulness of any definition (a math is a collection of definitions) is limited to the assumptions (givens) it makes.
I like math. But I often choose to abandon it. Because, I don't need to know the price of gas, or even the price to fill up. I will fill up the tank and pay whatever I am charged, because I have to hurry up and go to work so I can fill up the tank. If I am spending too much on gas, I don't need to do any accounting to know it. It's rather obvious. I don't need *precession* to deal with everyday matters.
Now, lets do some math. A fine rule of thumb is that when making decisions, we consider cost vs. benefit. Yep, but that's not a math, that's a guideline that can occasionally be used with a math. Here's a common type of problem. Say I have a $30 per month Internet subscription, and I have 100 e-mail addresses in my personal contact boon. The company offers me $20 off of one months fee, if I give them 5 e-mail address not already in their database.
Can you help? Can you even give me the name of the math that shows us how to convert between integrity and dollars?
We need pure mathematicans to develop new maths -- the lucky ones will get some recognition. But applied mathematicans are disposable. Let see what do we need them for? Well, they can weigh how much fuel was dispersed to a customer. (No, the pump already does that). Well, then obviously, they can figure the total price and required tax, and make change for the customers purchase! (No, No. The computers do all that. In fact, most people just swipe a credit card, the don't need change.) Well, then, we must need thew to setup the computers!
Society continues to elminate applied mathemeticans. The once was a need for computer programmers, and that need was unquestionable because people answered the call in droves. Now programmers are a dime a dozen. Which is good, because society screams do more, do more! So we programmed the machines and languages to do more. Have you seen Game Maker? Sweet, only about 30 hours to make the best game I've made to date. What's the problem then? Most the game programmers are now artists, not mathematically inclined. EA had, I believe, a 4:1 ratio between Graphics designers and core programmers working on The Sims 3. Why? Because society pays more pretty pictures than they do precise, concise code. See, the mathemeticians do their job so well, they eliminate their job. Made a cash register. Cool, now the book keeper only has to work 1 day a month. Made hard drives, made them bigger and faster, now we dont need efficient code, we don't have to pay for the best programmers, anybody that can click and drag will do.
I am not speaking against progress. I simply disagree. If there were a need for mathematicans, society would reward them better. The pure mathematicians typically teach, as they work on their own time to break new ground. It's a gamble that rarely pans out. The applied mathematicians do come and go as society dictates, ensuring a mediocre pay. But the time for them to go often comes sooner than they would like. Again, it's a very risky proposal.
There's no truth in math. There is a purpose, which is to create useful truths for specific situations. Math attempts to be more general, but it cannot be completely universal. (Godel) Which is why I personally don't but much stock in math, despite how much I enjoy it.
Well, at least I kept it short.
Sorry for typos, I switched keyboard layouts :(
A good example for useful geometry is construction. A former boss didn't carry a square. He used a tape measure and the 3,4,5 triangle to make 90 degree angles.
You're right that cost vs benefit is really economics. It's economics applying mathematical tools.
A pure mathematician is basically an academic. Study for the sake of study doesn't tend to pay well, it's not immediately productive. The same is true of Physics or other sciences. It doesn't change the fact that the engineers that design and build new technologies require the advance of scientific progress and scientific advances tend to require mathematial leaps. Newton is a great example, he was one of the people who had to develop calculus to solve general physical problems. At a much higher and much more specialised level the same still happens today, and still there are only handful of people in the world good enough to push the boundaries of pure maths.
Teachers, nurses, soldiers... all badly paid, does that mean they aren't needed?
Interestingly today there's a story that games makers are struggling to find enough qualified programmers with good maths knowledge.
http://news.bbc.co.uk/1/hi/technology/7460870.stm
Post a Comment